
So, let's formulate a piecewise linear regression model for these data, in which there are two pieces connected at x = 70: All you have to do is to enter functions with domains that line up. General method: The trick to this is knowing that expressions involving. Note: Piece wise functions are little pieces of. As you can see, the estimated two-piece function, connected at 70% -the dashed line -appears to do a much better job of describing the trend in the data. The graph tool automatically detects and graphs piecewise defined functions. Using a calculator to create the graph of a piecewise-defined function (TI 82). Select GRAPH mode from the main menu by using the arrow keys to highlight the GRAPH icon or pressing 5.
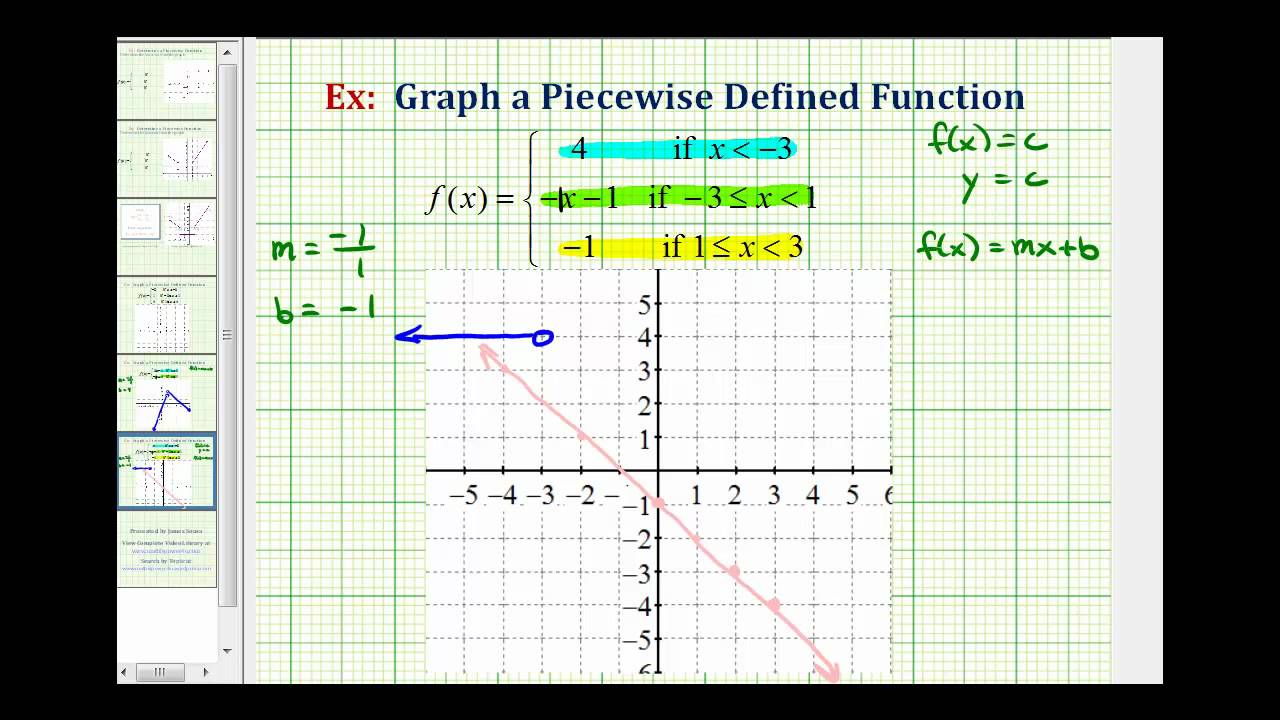
We could instead split our original scatter plot into two pieces -where the water-cement ratio is 70% -and fit two separate, but connected lines, one for each piece. Lets put the highlighted pieces together to get the graph of f(x). Provides yet more evidence that our model needs work. We use a closed circle for endpoints of graphs of piecewise functions when we have or.


 0 kommentar(er)
0 kommentar(er)
